The “Woolhouse method” or “Woolhouse approximation” is a well-known method for simplifying the evaluation of annuities that are paid more frequently than annually. A plethora of information about Woolhouse is available on the internet, but here is an elegant demonstration of this method.
Assume that the following are linear functions of 𝑡 for integral age 𝑥 and 0 ≤ 𝑡 ≤ 1:
|
• |
|
obviously |
|
• |
|
this is the UDD assumption |
|
• |
|
this is the Balducci hypothesis |
It follows that
if 
, 
, and 
are linear
functions of 𝑡,
then 
, 
, and 
are linear functions of 𝑡 as well. This produces
the 
shortcut.
A proof of this method is provided
below.
Proof of the 
shortcut
Assuming that is a linear
function of 𝑡 for
integral age 𝑥 and 0 ≤ 𝑡 ≤ 1, we evaluate using a straight line interpolation between 
and 
, hence:
for
integral 𝑥 and
0 ≤ 𝑡 ≤ 1
Since 𝑣00𝑝𝑥 =
1, this equation is simplified to:
Substituting 
for 𝑡 in the above
formula, we get the following formula which will be used in Formula 1
for this proof:
|
|
Formula
2 |
The present value of a life annuity can then be expressed as:
where 𝑃𝑉𝑌𝑒𝑎𝑟𝑥 is the present value at age 𝑥 of the 𝑚 payments to be paid during the year, and
Because we have linear for 0 ≤ 𝑡 ≤ 1,
then the 𝑚 payments are an arithmetic series, where the
sum of an arithmetic series is found by multiplying
the number of terms times the average of the first and last terms.
𝑃𝑉𝑌𝑒𝑎𝑟𝑥 is summed as follows:
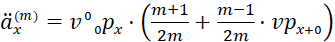
By substituting using Formula 2, this becomes:
And if we simplify and rearrange terms we get:
Then we have:
|
|
Formula 2.1 |
Which can be simplified to obtain the desired result:
|
|
Formula
2.2 |
|
|
Formula
2.3 |
|
|
Formula
2.4 |
|
|
Formula
2.5 |
Using this method, we have an 𝑚 times
speed improvement, because we need only calculate the present value of an
annuity with annual payments and then make the (𝑚 – 1)/2𝑚 adjustment.
Note that if an
annuity is temporary or deferred, or if the interest rate changes from one year
to the next, then we do not have the convenient simplification happening when
we go from Formula 2.1 to Formula 2.2!
For example:
·
for a 𝑛-year deferred life annuity
with payments made 𝑚 times per year, it
can be shown that:
·
for a 𝑛-year temporary life annuity with payments made 𝑚 times per
year, it can be shown that:
